Beer is best served cold, and math seems to help with that. Cláudio de Castro Pellegrini of the Federal University of São João del-Rei in Brazil recently set out to formulate an equation to determine the optimal form of one. the beer he was looking for a glass that will keep a drink sweetly chilled—that is, a glass that prevents the liquid in it from absorbing heat.
The easiest approach would be to find a container whose surface area is as small as possible relative to its volume. Therefore, the heat around the glass is absorbed into the surface of the glass. The smaller the surface, the less heat will enter, and the beer will be chilled for a longer time. Since ancient times, scholars recognized that in the case of two dimensions, a circle provides the minimum ratio of circumference to area. And this discovery also applies in three dimensions: a sphere has the smallest possible surface compared to its volume.
But a spherical beer glass would be impossible. What’s more, Pellegrini was not interested in a static situation that you leave behind beer in a glass and watch the liquid heat up. “The process here is quite simple: a beer is ordered, the waiter delivers it, it is served, it is consumed. Repeat.” he wrote in a preprint paper describing the researchwhich was published on the arXiv.org server in October. This means that the level inside the glass changes, as well as the surface in contact with the environment. (As more of the glass is drained, more of its surface comes in contact with the surrounding air.)
About supporting science journalism
If you like this article, please consider supporting our award-winning journalism subscribing. By purchasing a subscription, you’re helping to ensure a future of impactful stories about the discoveries and ideas that shape our world.
Pellegrini started with some limitations on the shape of the glass. It should consist of a flat, insulating base that is large enough to stand the glass. In addition, the glass must be symmetrical so that its shape can be represented by a body of revolution, or a three-dimensional shape created by rotating a two-dimensional one around 360 degrees. x axis or y axis To determine the optimal shape of the beer glass, Pellegrini adapted the 2D shape that defines the body of the revolution. In a previously printed studydetailed on the website arXiv.org in February, described optimal glass shapes as such, but the most viable containers had a volume of more than two liters—and the largest glass was more than 100 liters.
Next, Pellegrini aimed to design a realistic beer glass. But he had to make some simplifications. He assumed the base would insulate the heat perfectly. (In reality, a thick glass base and the use of coasters help, but perfect insulation is impossible.) He also assumed that the temperature of the beer in the liquid would be the same everywhere and that the drink would have a uniform density, ie. this is mostly true for filtered varieties. Pellegrini ignored beer foam as a possible insulating layer. And finally, ignoring the heat transfer from a person’s hand to the glass, he only investigated the effects of room temperature. “In the most extreme scenario, for example on a windy day at the beach (38 degrees Celsius), less than 3 minutes can be enough (again based on personal experience, completely replicated) to render beer undrinkable,” Pellegrini. he wrote
The Formula for the Optimal Beer Glass
With these assumptions, the researcher created an equation that describes the change in beer temperature over time. This is a differential equation, meaning the formula contains a derivative: a function that shows the rate of change of a variable (temperature in this case), which in this case is the shape of the beer glass:

here, T is the temperature of the beer, Tu is room temperature, cp is the specific heat capacity (how difficult it is to heat a substance), R is the density, v is the volume Ato is the exposed surface of the beer (including the side and the circular top surface) and hCV it is convective heat transfer, which indicates its ability to conduct heat. Using that formula, Pellegrini calculated four tips to make sure the beer cools as slowly as possible.
Ideally, you should enjoy your beer in a cool place like this Tu is as low as possible.
If you use a more insulating material such as ceramic instead of glass, the heat conduction coefficient decreases.
In general, Pellegrini points out that a thick head of foam insulates the beer and protects it from losing carbon dioxide too quickly. Furthermore, wind and drafts must be avoided, as these cause forced convection, which plays a much greater role in heat transfer than the natural convection considered here.
All of these tips can usually only be followed by moving to another location. If you’re sitting comfortably in a bar, you’re exposed to local conditions, so it would be welcome from the outset for bars to use glasses with a shape optimized for minimal heat exchange. Spoiler: it’s not.
The Beer Champagne Flute
The shape of the beer glass is captured in the quotient Ato⁄v in the above equation. The smaller the number, the smaller the temperature change. Therefore, he focused on reducing the value of Pellegrini Ato⁄v.
In school, mathematicians learn that these optimization problems can be solved with derivatives. For example, to find the vertex of the parabola f(x) = x2 + 3x + 2, the function is derived x and the result is set to zero: 0 = 2x + 3. This means that the vertex can be found here x = –1.5.
Pellegrini continued in the same way: he took out the quotient Ato/vset to zero and then use the properties of bodies of revolution to derive a function for the optimal shape of a beer glass. According to this, the radius R according to the height of a perfect beer glass h is given by this formula:

C is a constant, and Rb is the radius of the glass base. The formula shows that the opening of the mouth of the glass should be wide and the glass should be narrower towards the bottom, similar to many ordinary beer glasses. The radius of real-world glasses is not always progressively smaller, they often have a curved S shape when viewed from the side. This separates them from a perfect glass of beer.

The exact shape of the optimal glass depends on the radius and constant of the glass base C, which is determined by the height of the glass, among other things. Pellegrini first calculated the optimal shapes of glasses 19 centimeters high for different radii. As a result, it is reminiscent of a flat champagne.
The engineer then adapted the parameters of the perfect glass to different types of beer glass, such as the Imperial pint, the American pint or the Weizen (wheat beer) glass, which are widely used in the UK. base radius and height in its formula to calculate the optimal shape.
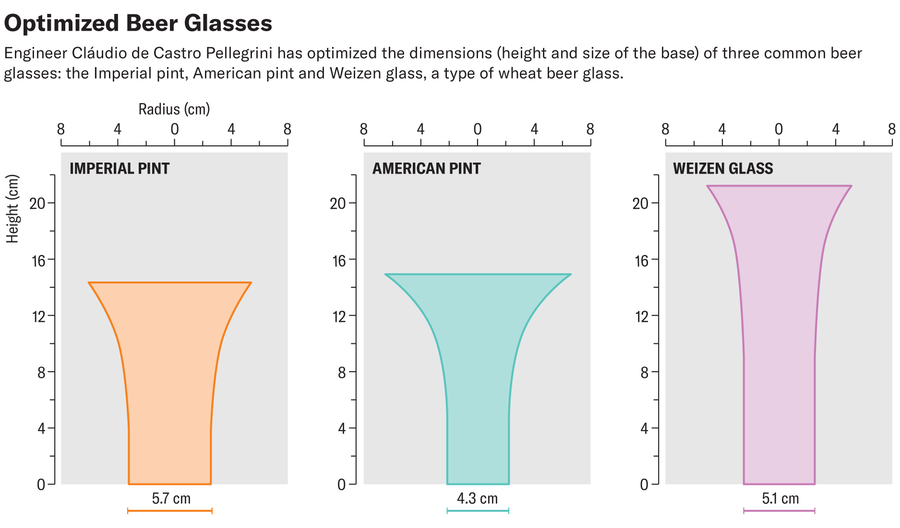
Here too the rough shape looks the same for all types and is reminiscent of a champagne flute. But even less-than-optimal glasses have some benefits, Pellegrini wrote in the preprint. Although the Nadir Figueiredo glass, which is widespread in Brazil, is far from the optimal shape, they are perfectly designed to transport heat in some respects: because of their relatively small beer volume, people usually drink beer faster than they can. heating up significantly.
This article originally appeared the spectrum of science and reproduced with permission.

